How to Find Principal Solution and General Solution

How to Find the General Solution of Trigonometric Equations?

Trigonometric Equations

Definition:
An equation involving one or more trigonometrical ratio of an unknown angle is called a trigonometrical equation

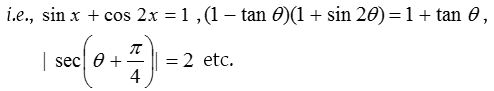
A trigonometric equation is different from a trigonometrical identities. An identity is satisfied for every value of the unknown angle e.g., cos² x = 1 − sin² x is true ∀ x ∈ R, while a trigonometric equation is satisfied for some particular values of the unknown angle.

(1) Roots of trigonometrical equation: The value of unknown angle (a variable quantity) which satisfies the given equation is called the root of an equation, e.g., cos θ = ½, the root is θ = 60° or θ = 300° because the equation is satisfied if we put θ = 60° or θ = 300°.

(2) Solution of trigonometrical equations: A value of the unknown angle which satisfies the trigonometrical equation is called its solution.
Since all trigonometrical ratios are periodic in nature, generally a trigonometrical equation has more than one solution or an infinite number of solutions. There are basically three types of solutions:

1. Particular solution: A specific value of unknown angle satisfying the equation.
2. Principal solution: Smallest numerical value of the unknown angle satisfying the equation (Numerically smallest particular solution).
3. General solution: Complete set of values of the unknown angle satisfying the equation. It contains all particular solutions as well as principal solutions.

Trigonometrical equations with their general solution

Trigonometrical equation	General solution
sin θ = 0	θ = nπ
cos θ = 0	θ = nπ + π/2
tan θ = 0	θ = nπ
sin θ = 1	θ = 2nπ + π/2
cos θ = 1	θ = 2nπ
sin θ = sin α	θ = nπ + (−1)nα
cos θ = cos α	θ = 2nπ ± α
tan θ = tan α	θ = nπ ± α
sin2 θ = sin2 α	θ = nπ ± α
tan2 θ = tan2 α	θ = nπ ± α
cos2 θ = cos2 α	θ = nπ ± α
sin θ = sin α cos θ = cos α	θ = nπ + α
sin θ = sin α tan θ = tan α	θ = nπ + α
tan θ = tan α cos θ = cos α	θ = nπ + α

General solution of the form a cos θ  +  b sin θ  = c

Method for finding principal value

Suppose we have to find the principal value of sin θ = −½  satisfying the equation .
Since sin θ is negative, θ will be in 3^rd or 4^th quadrant. We can approach 3rd or 4th quadrant from two directions. If we take anticlockwise direction the numerical value of the angle will be greater than π. If we approach it in clockwise direction the angle will be numerically less than π. For principal value, we have to take numerically smallest angle. So for principal value.
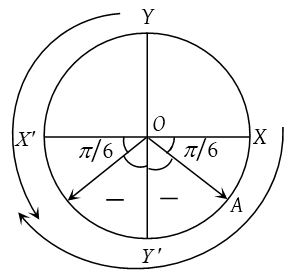(1) If the angle is in 1st or 2nd quadrant we must select anticlockwise direction and if the angle is in 3^rd or 4^th quadrant, we must select clockwise direction.
(2) Principal value is never numerically greater than π.
(3) Principal value always lies in the first circle (i.e., in first rotation). On the above criteria, θ will be −π/6 or −5π/6 Between these two −π/6 has the least numerical value. Hence −π/6 is the principal value of θ satisfying the equation sin θ = −½.
From the above discussion, the method for finding principal value can be summed up as follows :

1. First draw a trigonometrical circle and mark the quadrant, in which the angle may lie.
2. Select anticlockwise direction for 1^st and 2^nd quadrants and select clockwise direction for 3^rd and 4^th quadrants.
3. Find the angle in the first rotation.
4. Select the numerically least angle. The angle thus found will be principal value.
5. In case, two angles one with positive sign and the other with negative sign qualify for the numerically least angle, then it is the convention to select the angle with positive sign as principal value.

Trigonometric Equations Problems with Solutions

1.

Solution:

2.

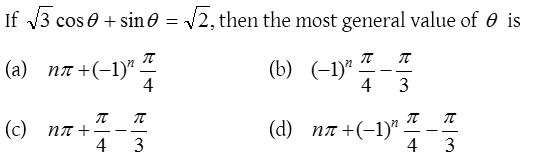
Solution:

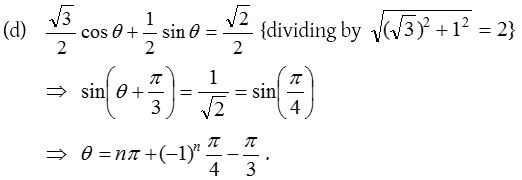
3.

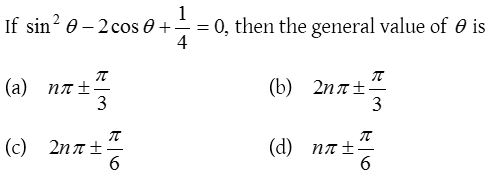
Solution:

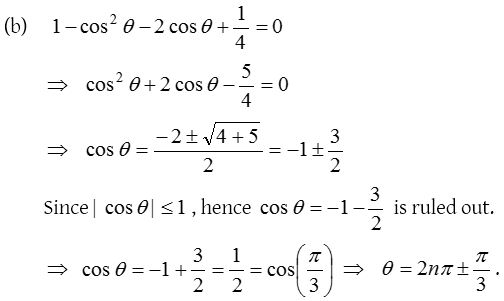
4.

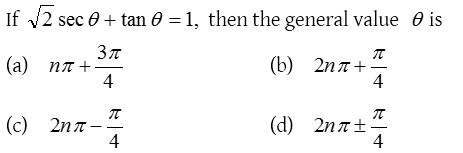
Solution:

5.

Solution:

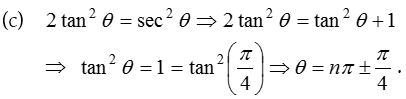
6.

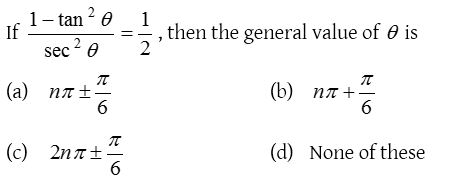
Solution:

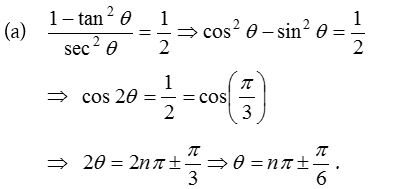
7.

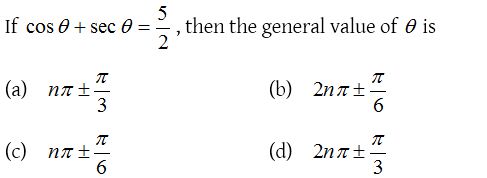
Solution:

8.

Solution:

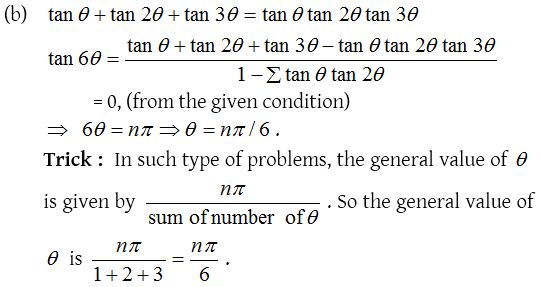
9.

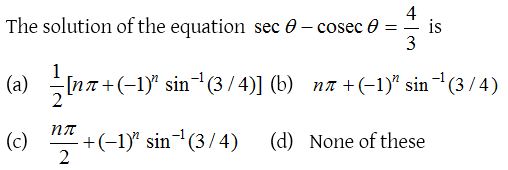
Solution:

Basic Trigonometric Equations:

When asked to solve 2x – 1 = 0, we can easily get 2x = 1 and x = as the answer.
When asked to solve 2 sin x – 1 = 0, we proceed in a similar manner. We first look at sin x as being the variable of the equation and solve as we did in the first example.
2 sin x – 1 = 0
2 sin x = 1
sin x = 1/2

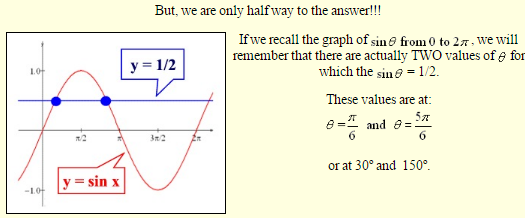
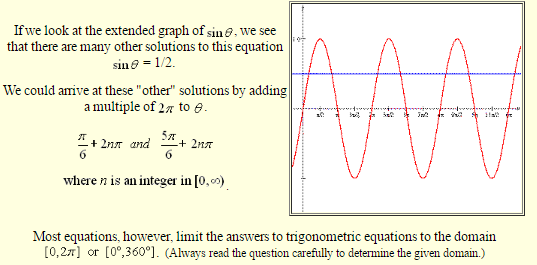
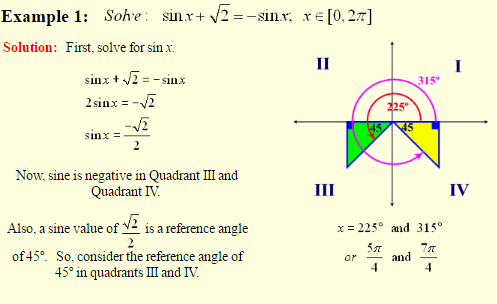
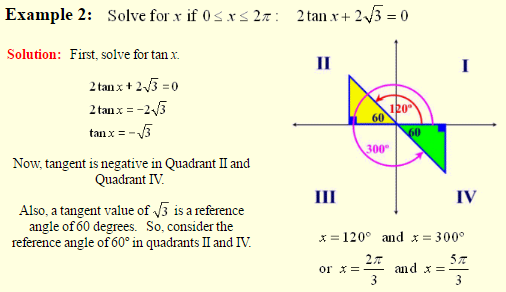
Signs and Quadrants:

Solutions of trigonometric equations may also be found by examining the sign of the trig value and determining the proper quadrant(s) for that value.

How to Find Principal Solution and General Solution

Source: https://www.aplustopper.com/trigonometric-equations/

Share :